Eratosthenes (l. c. 276-195 BCE) was a Greek astronomer, geographer, mathematician, and poet best known for being the first to calculate the circumference of the earth and its axial tilt. He is also recognized for his mathematical innovation, the Sieve of Eratosthenes, which identified prime numbers, and his position as head of the Library at Alexandria.
He was a native of Cyrene, North Africa (modern-day Shahhat, Libya), a prosperous Greek center of trade on the coast of the Mediterranean Sea. As a young man, he was sent to study in Athens at Plato's Academy under Arcesilaus (l. c. 316-240 BCE) who had instituted the system of Academic Skepticism at the school. Academic Skepticism encouraged people to question what constituted "knowledge" and to test accepted conclusions about the world themselves rather than repeating what others had claimed as truth. This course of study is thought to have influenced Eratosthenes' later work.
He was called to Alexandria, Egypt by Ptolemy III Euergetes (r. 246-222 BCE) as head curator of the famous library there. Eratosthenes remained in Alexandria for the rest of his life, and it was there he made his calculations regarding the circumference of the earth and wrote his best-known works, including a three-volume set on geography credited with coining that term. He was a friend of the inventor and mathematician Archimedes (287-212 BCE) and the two encouraged each other's work.
Whereas Archimedes focused on physics, astronomy, and engineering innovations, Eratosthenes engaged with everything that interested him. This earned him the nickname Beta (second letter of the Greek alphabet) for being second-best at everything though, at the same time, he was praised as highly as an Olympian victor for his various works. In his eighties, he began to lose his eyesight and, feeling he had no reason to live any longer, starved himself to death. He is remembered as one of the greatest polymaths of antiquity and many of the conclusions he reached and terms he coined are still used today.
Early Life & Education
Eratosthenes was born, the son of one Aglaos, in Cyrene which had been established as a Greek colony in North Africa in 631 BCE. It quickly became a prosperous port of trade, establishing itself as a republic by the mid-5th century BCE, until it was taken by Alexander the Great. After Alexander's death in 323 BCE, it was ruled by the Ptolemaic Dynasty of Egypt and, in the year of Eratosthenes' birth, declared its independence. It was brought back under Ptolemaic control in 246 BCE by Ptolemy III, when Eratosthenes was still in Athens where his father had sent him to receive an education.
Athens, at that time, was the intellectual center of the Mediterranean and Plato's Academy one of the most prestigious educational institutions. Eratosthenes studied under Arcesilaus, a Skeptic philosopher, who encouraged the philosophical discipline of Skepticism first articulated by Pyrrho of Elis (l. c. 360 to c. 270 BCE). Pyrrho wrote nothing himself, but his system was preserved by his student Timon of Phlius (l. c. 320 to c. 235 BCE) and developed by others until Arcesilaus instituted its tenets as the guiding principle of the Academy.
Pyrrho's philosophy maintained that people were troubled in life by trusting sense perceptions and believing that conclusions based upon them constituted reality. It is unclear whether Pyrrho claimed that objective reality was unknowable or simply that humans lacked the capacity to know it but, either way, the practical outcome was the same: one's sense perceptions were incapable of rendering the truth about observable phenomena and so, to achieve peace of mind, one should keep from making any judgments or coming to any firm conclusions regarding the sensible world.
The best one could do, Pyrrho would suggest, was to remain uncommitted to any kind of conclusion, balancing reasons for and against accepting it equally, and leaving it at that. By doing so, one would achieve a state of mind known as ataraxia, freedom from psychological distress and personal upset. By the time of Arcesilaus, Pyrrho's philosophy had developed to center on the claim that knowledge was impossible because humans were incapable of apprehending the sensible world through sense perceptions. Since one could not know what one did not know, and what one did know was limited to unreliable sense perceptions, one would never be able to find out what one did not know or whether what one knew was, in fact, true.
Eratosthenes & Skepticism
There is no record of Eratosthenes' time in Athens or what he would have studied but he would have been exposed to this philosophical model at the Academy. Although he is not regarded as a skeptic philosopher, his later work suggests he followed the essential skeptic paradigm of refusing to accept the conclusions of others, rejecting the impressions of sense perceptions, and attempting to find the truth about any given subject through reasoned applications. The later skeptic philosopher and compiler, Sextus Empiricus (l. c. 160 to c. 210 CE) defines the skeptic principles which seem to have informed Eratosthenes' views and methodology:
The originating cause of skepticism is, we say, the hope of attaining quietude. Men of talent, who were perturbed by the contradictions in things and in doubt as to which of the alternatives they ought to accept, were led on to inquire what is true in things and what is false, hoping by the settlement of this question to attain quietude. The main basic principle of the skeptic system is that of opposing to every proposition an equal proposition; for we believe that as a consequence of this we end by ceasing to dogmatize. (I.VI.12)
Accepting dogmas as truths, skepticism claimed, locked one into an established mindset which one then felt compelled to defend instead of question. By remaining skeptical of all dogma, one was free to explore the subject matter on one's own through reason. By pursuing this course, one might still not come to know what one did not know but would at least know that what one knew could be considered accurate. This seems to have been the model that influenced Eratosthenes' inquiries into accepted knowledge of his time.
While still living and studying in Athens, Eratosthenes wrote a number of works, now lost, which were cited by later writers and suggest an application of skeptic principles. Among these was a series of histories, the Chronographies, recalculating important dates in history beginning with the Trojan War, which was highly regarded as more accurate than previous works. Another of his works dealt with the mathematical aspects of Plato's philosophy, possibly demonstrating how philosophical claims could be proven mathematically, though this is speculation as the work has been lost. These works, and others, were brought to the attention of Ptolemy III Euergetes who requested he come to Alexandria to take over the operation of the library.
Alexandria & the Circumference of Earth
The Ptolemies were interested in making Alexandria the rival of Athens as an intellectual center and, to that end, had already built the library there close to the great temple of the god Serapis (the Serapeum). The Great Library at Alexandria, containing thousands of scrolls, was supplemented by a second in the Serapeum as Ptolemy III made the acquisition of books a priority. Every ship arriving at Alexandria's port was boarded and searched for books. Once found, they were copied, and the copies were returned to their owners; the originals became part of Alexandria's collection. It is said that these copies were so precise that one could not tell them apart from the originals.

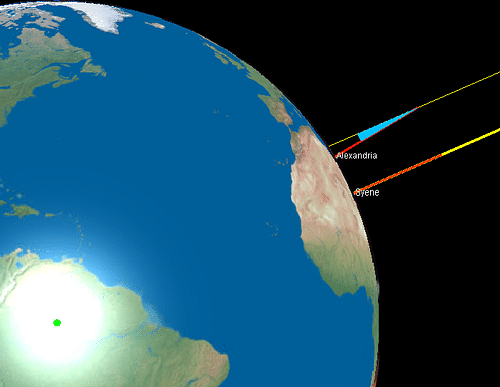
Eratosthenes, as head librarian, was responsible for the acquisition of these books and the quality of the copies and was also tutor to Ptolemy III's children. He took the Ptolemaic vision of Alexandria as a great seat of learning seriously, expanding the library's collection and organizing it into more clearly defined sections. While going about his various duties, he heard of a well in the city of Syene (modern-day Aswan) to the south whose water (not the sides of the well) was fully illuminated by the sun at noon on the summer solstice (around 21 June), suggesting the sun was directly overhead. He observed that, on the same day, obelisks and other objects in Alexandria cast long shadows and understood that, by calculating the distance between the two cities and the angle of the sun, he could find the circumference of the earth.
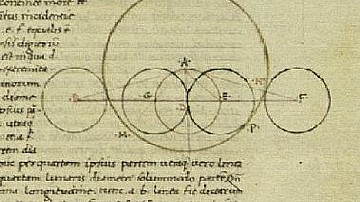
In c. 240 BCE, he erected a pole at Alexandria and hired a man to walk the distance from Alexandria to Syene to measure the distance (though it was already known from trade caravans). Once he knew the distance was 5,000 stadia (500 miles/800 km), he measured the angle of the sun's rays by this pole (dividing the length of the shadow by the height of the pole) for an angle of 7.12 degrees. The Greeks already knew the earth was round and regarded it as a circle of 360 degrees and so by dividing 360 by 7.2 (so that 360 would divide evenly) he arrived at a circumference for the earth of 250.000 stades (approximately 24,854 miles/40,000 km). Eratosthenes published his conclusions in his On the Measure of the Earth which only exists today as fragments in the works of other writers, beginning with the astronomer Cleomedes whose text is the basis for those that followed.
His calculations were generally accepted as accurate and the geographer Strabo (l. 64 BCE to c. 24 CE), in his Geography, notes that, while this was not true of everyone later on, Eratosthenes' calculations continued to be regarded as sound and were still in use at the time of the astronomer Hipparchus of Nicea (190-120 BCE):
Now, his introduction of the principles of mathematics and physics into the subject [of geography] is a commendable thing; also his remark that if the earth is sphere-shaped, just as the universe is, it is inhabited all the way round; and his other remarks of this nature [as well]. But as to the question whether the earth is as large as he has said, later writers do not agree with him; neither do they approve his measurement of the earth. Still, when Hipparchus plots the celestial phenomena for the several inhabited places, he uses, in addition, those intervals measured by Eratosthenes on the meridian through Meroe and Alexandria and the Borysthenes, after saying that they deviate but slightly from the truth. (Book I.4.1)
Strabo was commenting here on Eratosthenes' 3-Volume work Geography which sought to accurately map and chart the world. His first volume criticized Homer's Odyssey as geographically absurd in that the various lands Odysseus is said to have visited were much closer to each other than depicted. This volume also coined the term geography and provided an introduction to the subject. The second volume contained, among other observations, the steps he took in calculating the circumference of the earth, and the third volume is said to have detailed the various inhabited lands and commented on the peoples found there.
Strabo, in commenting on the work, notes how Eratosthenes objects to the Greek sense of superiority over others, claiming all people should be judged on individual merits, not by race, ethnicity, or nationality:
Now, towards the end of his treatise – after withholding praise from those who divide the whole multitude of mankind into two groups, namely, Greeks and Barbarians, and also from those who advised Alexander to treat the Greeks as friends but the Barbarians as enemies – Eratosthenes goes on to say that it would be better to make such divisions according to good qualities and bad qualities; for not only are many of the Greeks bad, but many of the Barbarians are refined. (Book I.4.9)
Eratosthenes' Geography, like his other works, is also lost but was considered a significant intellectual achievement in antiquity as well as controversial since it challenged the Homeric view of the world which many claimed as irrefutable truth. In keeping with his skeptical education, however, Eratosthenes refused to accept the popular understanding of any subject and sought always to find out the truth of a matter for himself.

Sieve of Eratosthenes
An example of this is his algorithm known as the Sieve of Eratosthenes which located prime numbers. A prime number is any natural number that is not reached by combining two smaller numbers while a composite number is the product of any given smaller amounts. The Greeks did not define the number 1 even as a number and so the number 2 was understood as the first prime number. The Sieve of Eratosthenes was so-called because it acted like a sieve to separate prime numbers from composite numbers. Beginning with the number 2, the multiples of composite numbers are sequentially revealed until all that is left in the “sieve” are prime numbers. This innovation assisted in easier and more efficient mathematical calculations.
He is also said to have written works on drama and the theater in general, ethics, astronomy, and to have mapped the Nile River further and more accurately than anyone before him. He was also known to have created a calendar which accounted for leap years, but these contributions have all been lost. Scholar T. L. Heath comments:
In the work On the Measurement of the Earth, Eratosthenes is said to have discussed other astronomical matters, the distance of the tropic and polar circles, the sizes and distances of the sun and moon, total and partial eclipses, etc. (Livingstone, 127)
A true polymath, Eratosthenes is said to have contributed significantly to many different subject areas and disciplines before his failing eyesight led him to take his own life. Although he is said to have been given the nickname Beta – the equivalent of the phrase "jack of all trades, master of none" in the present day – his reputation for excellence in many different fields suggests this was more of a joke than anything to be taken seriously.
Conclusion
Though his work was highly regarded, it was still challenged and his calculations for the circumference of the earth were reworked by the later astronomer Posidonius of Rhodes (l. c. 135 to c. 51 BCE). Posidonius' system was considered easier to use and was closer to the dimensions proposed by Aristotle (384-322 BCE) who was considered the standard by which any claims were measured.
Posidonius' calculations resulted in a smaller circumference for the earth but, because they had become more popular than Eratosthenes', were the ones used in the bestselling work Almagest of the astronomer Ptolemy (100-170 CE) which would continue to exert considerable influence up through the European Renaissance. Although Eratosthenes' calculations were still in use, Posidonius' system was considered valid by virtue of its inclusion in Ptolemy's work, and it was Posidonius' calculations that Christopher Columbus (1451-1506) used to convince his patrons to sponsor him as he was able to show them how short a trip across the Atlantic Ocean would be.
Eratosthenes' system was more accurate, however, and was used by many European ship captains and cartographers effectively throughout the Age of Exploration. In the present day, Eratosthenes' calculations are understood as being closer to the actual circumference of the earth than either Posidonius or Aristotle and he is recognized as one of the greatest intellectuals of antiquity.