Archimedes (l. 287-212 BCE) was a Greek engineer and inventor who is regarded as the greatest mathematician of antiquity and one the greatest of all time. He is credited with a number of inventions still in use today (such as the Archimedes screw) and is referred to as the father of mathematics and mathematical physics.
He was born in the Greek colony of Syracuse, Sicily, and lived there all his life except for a brief time spent studying in Alexandria, Egypt, where he became friends with the polymath Eratosthenes (l. c. 276-195 BCE) and the astronomer Conon of Samos (l. c. 280 to c. 220 BCE). Upon returning to Syracuse, he worked for King Hiero II (r. 270-215 BCE), to whom he may have been related, as an engineer and problem-solver. He is said to have devised or improved upon a number of weapons for the defense of Syracuse against the Romans during the Second Punic War (218-201 BCE) including a heat ray whose existence and efficacy are still debated.
Archimedes is best known for his invention of the Archimedes screw, application of the lever, and his mathematical advances. He is said to have been so completely absorbed by intellectual pursuits that he would frequently forget to eat or bathe. This single-mindedness may have contributed to his death as, after the fall of Syracuse to the Romans in 212 BCE, he was ordered by a soldier to follow him but was absorbed in mathematical calculations and refused. He was then killed by the soldier, who did not recognize him, against the express orders of the Roman general Marcus Claudius Marcellus (l. c. 270-208 BCE). He was regarded as a mathematical and engineering genius in his time, and this reputation is maintained in the present day.
Life & Alexandria
Almost nothing is known of Archimedes' life except that he was born in Syracuse, Sicily, which was then part of the region known as Magna Graecia ("Greater Greece"), the Roman term for the areas settled by Greek colonization along the coast of Southern Italy. His father, an astronomer, was named Phidias, and it is thought his family was of the upper class, or possibly nobility, as they could afford to send him to Alexandria for an education. The first extant reference to Archimedes comes from the works of Polybius (l. c. 200 to 118 BCE), who was primarily interested in the war machines Archimedes designed. Polybius most likely omitted information on Archimedes' life because a biography (now lost) had already been published.
At some point, his father sent him to Alexandria which, at that time, was developing as an intellectual center, rivaling Athens, under the Ptolemaic Dynasty (323-30 BCE). In Alexandria, he became friends with Eratosthenes of Cyrene and Conon of Samos, both leading intellectuals of the city. Conon was a well-respected astronomer and mathematician, and Eratosthenes was head of the Library of Alexandria and a polymath who first calculated the circumference of the earth. The details of these relationships are unknown, but Archimedes admired Eratosthenes well enough to dedicate his work The Method to him.
Astronomical Advances
It is likely that both Conon and Eratosthenes influenced Archimedes in the disciplines of mathematics and astronomy, but any suggestion on how great that influence may have been is speculative. Archimedes is said to have written a number of works on astronomy, alluded to by later writers, but none of these have survived except his Sand-Reckoner, which calculated the size of the universe. The title comes from his attempt to determine how many grains of sand would fill the universe and, to do this, he needed to know how large the universe was. The work is best known for preserving the heliocentric model proposed by the astronomer Aristarchus of Samos (l. c. 310 to c. 230 BCE). Scholar T. L. Heath comments:
The Sandreckoner is remarkable for the development in it of a system for expressing very large numbers by orders and periods based on powers of myriad-myriads. It also contains the important reference to the heliocentric theory of the universe put forward by Aristarchus of Samos in a book of 'hypotheses', as well as historical details of previous attempts to measure the size of the earth and to give the sizes and distances of the sun and moon. (Livingstone, 125)
Archimedes is said to have invented astronomical devices which could identify the positions and motions of the sun, moon, and planets. At least one of these devices is described as a bronze sphere which, when turned, showed the planetary positions and how they revolved around the earth (as the earth was understood as the center of the universe at that time). This mention of the devices by the later writer and orator Cicero (l. 106-43 BCE) is cited by modern-day scholars as suggesting Archimedes as the most likely inventor of the Antikythera mechanism.
The Antikythera mechanism (also known as the Antikythera device) is believed to be the world's first analogue computer. The device, discovered in 1901 off the Greek island of Antikythera, dates to the late 2nd century/early 1st century BCE and was used to calculate the position of the sun, moon, and planets. The device relied on Babylonian and Egyptian astronomical principles but used letters from the Greek alphabet and was manufactured in Greece. By turning a crank, one moved a pointer, which clicked into place to show the phase of the moon, the location of the planets, and could also calculate an eclipse.
Archimedes is only one contender as the inventor of the device as it has also been attributed to Hipparchus of Nicea (l. 190-120 BCE) and others. Cicero's mention of Archimedes' similar inventions, however, is corroborated by the mathematician Pappus of Alexandria (l. 290 to c. 350 CE), who claimed that Archimedes had written a work on how to construct such devices. This does not mean, however, that Archimedes built the Antikythera device – his works may have inspired Hipparchus or someone else in its creation – and the identity of the inventor continues to be debated.
Archimedes' Screw
Whether he was the creator of the Antikythera device, he is well-established as the inventor of the Archimedes screw, a means of drawing water from a lower level up to a higher one. As with many of the stories concerning Archimedes' life, there are variations on the details of the circumstances leading to the creation of Archimedes' screw, but they all involve the problem of removing water from the lowest deck of a ship.
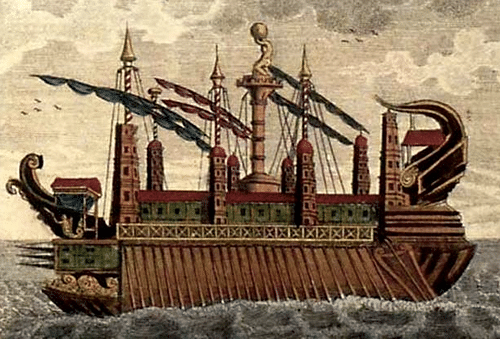
The best-known version comes from the Greek writer Athenaeus of Naucratis, who relates how Hiero II requested Archimedes design a massive ship for him, the greatest anyone had ever seen, which could serve in shipping, as a luxury vessel, or for warfare. Archimedes designed the largest ship ever built, the Syracusia, which featured an elaborate temple to Aphrodite, gardens, a gym, state rooms, and other amenities, room enough for over 1900 passengers, crew, and soldiers, and war towers as well as a full-sized catapult on board. The ship was built according to Archimedes' plans but then, because of its size and weight, was found to leak a considerable amount of water through its hull.
Archimedes' screw was a cylinder enclosing a twisted blade that revolved upwards when turned by a crank. By placing one end of the cylinder in the water and turning the crank, water would be drawn up and removed from the ship. This mechanism is still used today in a number of applications around the world. The Syracusia sailed only once, from Syracuse to Alexandria, where it was presented as a gift to Ptolemy III Euergetes (r. 246-222 BCE), but what happened to it after that is unknown.
Archimedes' Principle
The ship is featured in some versions of the story of Archimedes' principle which established that any floating object displaces its own weight of the fluid it is in. Archimedes' principle explains why an object floats instead of sinking (the principle of flotation), and so he is known as the father of hydrostatics. It is said that Archimedes arrived at his conclusions in trying to work out how a ship as large as the Syracusia would be able to float. Archimedes' text, On Floating Bodies (still extant) never mentions the Syracusia in regard to his discovery, but neither does he mention the famous golden crown which features in most versions of the story.
According to the best-known version, by the Roman architect and engineer Vitruvius (l. c. 90 to c. 20 BCE), Hiero II supplied an artisan with pure gold to make a crown. When the crown was presented to the king, he suspected the goldsmith had used a baser metal and only plated it with some of the gold, keeping most of it. He asked Archimedes to devise some method of discovery without damaging the crown. Archimedes is said to have thought on this problem for some time until one day, lowering himself into his bath, he noticed the water rose as it was displaced by his body and understood this principle could be used in establishing the density of the crown. He is said to have become so excited at this revelation that he ran out of his house and down the street naked, yelling "Eureka!" ("I have found it!"). Heath elaborates on the principle:
Archimedes invented the whole science of hydrostatics. Beginning the treatise On Floating Bodies with an assumption about uniform pressure in a fluid, he first proves that the surface of a fluid at rest is a sphere with its center at the center of the earth. Other propositions show that, if a solid floats in a fluid, the weight of the solid is equal to that of the fluid displaced, and, if a solid heavier than a fluid is weighed in it, it will be lighter than its true weight by the weight of the fluid displaced. Then, after a second assumption that bodies which are forced upwards in a flued are forced upwards along the perpendiculars to the surface which pass through their centers of gravity, Archimedes deals with the position of rest and stability of a segment of a sphere floating gin a fluid with its base entirely above or entirely below the surface. Book II is an extraordinary tour de force, investigating fully all the positions of rest and stability of a right segment of a paraboloid floating in a fluid according, (1) to the relation between the axis of the solid and the parameter of the generating parabola and, (2) to the specific gravity of the solid in relation to the fluid; the term 'specific gravity' is not used, but the idea is fully expressed in other words. (Livingstone, 125)
According to Vitruvius, Archimedes used this principle to determine the density of the crown and found that the goldsmith had indeed used baser metal and kept most of the gold for himself.
Mathematical Advances
Archimedes' fame as a mathematical genius rests on a number of his works, many still extant, which are considered major contributions to the field. Heath writes:
Archimedes' works are all original and are perfect models of mathematical exposition; their wide range will be seen from the list of those which survive: On the Sphere and Cylinder I, II, Measurement of a circle, on Conoids and Spheroids, on Spirals, on Plane Equilibriums I, II, The Sandreckoner, Quadrature of the Parabola, On Floating Bodies I, II, and lastly, The Method, only discovered in 1906. (Livingstone, 123)
The last work Heath references is The Method of Mechanical Theorems which was only identified as a work of Archimedes in the original Greek in 1906 by the historian, philologist, and Archimedes specialist Johan Heiberg (l. 1854-1928). It was hidden behind a later text of a Christian liturgical work that had reused the pages of the older piece. It was a common practice in the Middle Ages to recycle older books by unbinding them, scraping off the pages and washing them, and then using them for a new piece, because vellum parchment was costly.
The work Heiberg identified (now known as the Archimedes Palimpsest) was reused in this way in around 1229 with the liturgical text written over the faded original, which, even so, could still be read. Recent work with imaging between 1999-2008 has made Archimedes' work clearer and The Method is now able to be read as a complete work.
Archimedes calculated the value of Pi as 3.14, established calculus through his discovery of infinitesimals, defined parabolas, formulated the area of a circle, and described the property of real numbers, among other major contributions. His work was not purely speculative or abstract thought, however, as he applied mathematics to problem-solving and design as in the case of his famous war machines.
War Machines
Archimedes is often credited with inventing the lever but what he actually did was explain how the lever worked and allow for more precise use of it. His invention of the odometer (which measures distance) used the lever on a cart that, when rolled, deposited a small ball to mark each mile between two points. The lever was also used to improve existing catapults for the defense of Syracuse against the Romans.
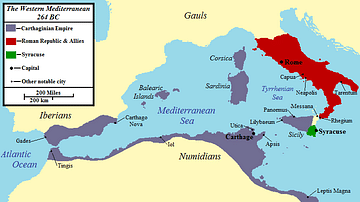
During the Second Punic War, Syracuse was allied with Rome but switched sides to support Carthage. Rome sent the generals Claudius Marcellus and Appius Claudius Pulcher (d. 211 BCE) against Syracuse in 214 BCE to bring it back in line. The Romans seem to have thought the campaign would be an easy one until they encountered Archimedes' war machines which are said to have defended the city for two years. Aside from the improved catapults, the two best-known devices were the claw of Archimedes and his heat ray.
The claw of Archimedes was a crane-like mechanism with a hook at one end which could be deployed to destroy ships. There seems to have been more than one of these devices deployed seaward, and when Roman ships drew near, the crane would swing down and either capsize the ship or pick it up to dash it into another. The Roman historian Livy (l. 59 BCE to 17 CE) notes that Rome suffered heavy losses due to Archimedes' defenses and remarks on the efficacy of the claw device specifically. Modern-day reconstructions of the claw of Archimedes have proven that the device probably worked as described by ancient historians.
The heat ray is far more controversial in the present day as some scholars continue to question how it worked or if it even existed. The device is never defined in any of the works that mention it. The writer Lucian of Samosata (l. c. 125 to c. 180 CE), for example, only says that Archimedes destroyed enemy ships with fire, and Anthemius of Tralles claims he did so using "burning-glasses," but neither describes how this was done.
The 12th-century writer John Tzetzes of Byzantium (l. c. 1110-1180), however, provides the most detailed description in his Chiliades (Histories), Book II.119-127:
The old man [Archimedes] constructed some sort of six-angled mirror. Having set from an equally measured interval of the mirror small such mirrors, fourfold in their corners, that were set in motion both by cups and some hinge joints, he set that six-angled mirror in the middle of rays of the sun when it was midday both in the summer and in most of the wintry season. When the rays, later, were reflected into this, a fearful fiery kindling was lifted to the vessels, and reduced them to ashes from the length of a bow shot. (Tzetzes, Chiliades, 2)
This account is taken from a lost work by the mathematician Pappus of Alexandria (l. c. 290 to c. 350 CE). The practicability of the heat ray as described has been tested in the modern day and found to be implausible, but it is unclear how accurate Pappus' description was or how the mirrors were angled. Scholars continue to debate whether the device ever existed as described, but it seems some sort of invention – not a catapult – was created by Archimedes that set ships on fire from a distance.
Conclusion
Archimedes' defenses, whatever they were, effectively held off the Romans for two years until they breached the outer walls of the city while the defenders were distracted by preparations for a religious festival honoring Artemis. Marcellus gave strict orders that Archimedes was to be taken alive as he seems to have known he was behind the success of the city's defenses and considered him a military asset.
According to Plutarch (l. c. 45/50 to c. 120/125 CE), Archimedes was engaged in some calculations on the beach when he was approached by a Roman soldier who summoned him to follow. Archimedes was so deeply engaged with whatever he was doing he allegedly told the man, "Do not disturb my circles," referring to the diagrams he had drawn in the sand. These are said to have been his last words as the soldier, not recognizing him, drew his sword and killed him. It has also been suggested that the soldier knew who he was and killed him to avenge the many Romans who had died from his inventions.
He was buried in an elaborate tomb in the city, but how this would have happened is unclear since Marcellus lay siege to the inner citadel for another eight months after Archimedes' death and then completely sacked the city. Cicero, however, claims to have visited the tomb, which was in a state of neglect and decay, and he had it restored when he was serving as a magistrate in Sicily. The tomb is said to have been ornamented with a sculpture of a sphere and a cylinder, the focus of Archimedes' famous work of that name, honoring one of the greatest minds of antiquity.